Posts Tagged: philosophy

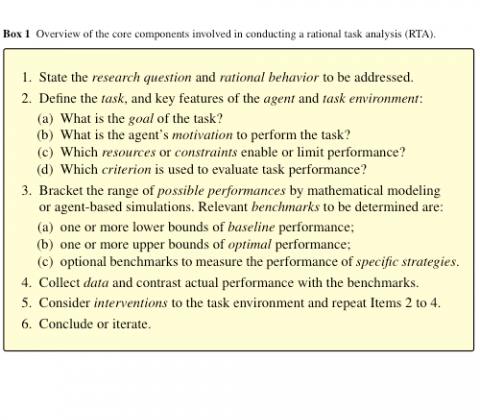
Paper: Rational task analysis (RTA)
a theory of thinking and problem solving cannot predict behavior
unless it encompasses both an analysis of the structure of task environments
and an analysis of the limits of rational adaptation to task requirements.
(Newell & Simon, 1972, p. 55)
Hansjörg Neth, Chris R. Sims, Wayne D. Gray
Rational task analysis: A methodology to benchmark bounded rationality
Abstract: How can we study bounded rationality? We answer this question by proposing rational task analysis (RTA)—a systematic approach that prevents experimental researchers from drawing premature conclusions regarding the (ir-)rationality of agents. RTA is a methodology and perspective that is anchored in the notion of bounded rationality and aids in the unbiased interpretation of results and the design of more conclusive experimental paradigms.

Chapter: The cognitive basis of arithmetic
| The truths about numbers are in us; but still we learn them. |
| G.W. Leibniz (1765), Nouveaux essais sur l’entendement humain, p. 85 |
Helen De Cruz, Hansjörg Neth, Dirk Schlimm
The cognitive basis of arithmetic
Overview: Arithmetic is the theory of the natural numbers and one of the oldest areas of mathematics. Since almost all other mathematical theories make use of numbers in some way or other, arithmetic is also one of the most fundamental theories of mathematics. But numbers are not just abstract entities that are subject to mathematical ruminations — they are represented, used, embodied, and manipulated in order to achieve many different goals, e.g., to count or denote the size of a collection of objects, to trade goods, to balance bank accounts, or to play the lottery. Consequently, numbers are both abstract and intimately connected to language and to our interactions with the world. In the present paper we provide an overview of research that has addressed the question of how animals and humans learn, represent, and process numbers.
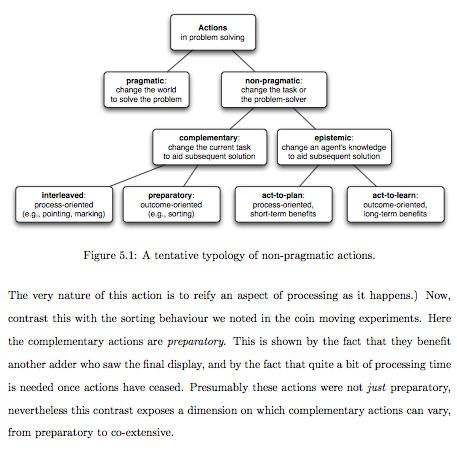
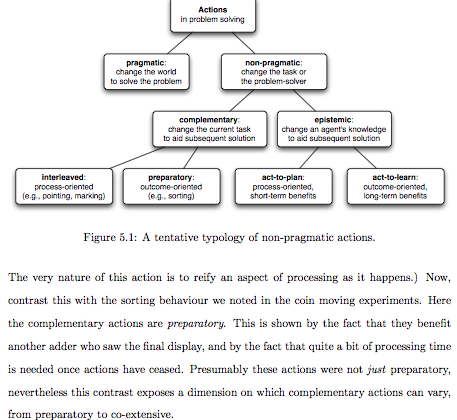
Paper: A taxonomy of (practical vs. theoretical) actions
| The solution to a problem changes the problem. |
| Peer’s Law |
Hansjörg Neth, Thomas Müller
Thinking by doing and doing by thinking: A taxonomy of actions
Abstract: Taking a lead from existing typologies of actions in the philosophical and cognitive science literatures, we present a novel taxonomy of actions.